Medians of Triangles
By: Lacy Gainey
The CENTROID (G) of a triangle is the point of concurrency of the three medians.
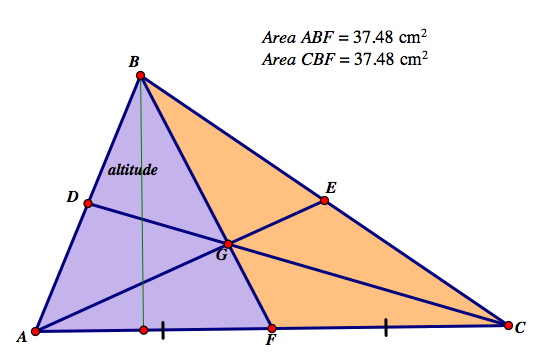
A median of a triangle is the segment from a vertex to the midpoint of the opposite side. The medians divide the triangle into six small triangles.
We want to show that these triangles all have the same area.
Observe the triangle below,

We know that AF≅ CF, CE ≅ BE, and BD ≅AD.
Since we do not know the measurements of AG, DG, BG, EG, CG, and FG, it would be difficult to start out proving that one of the six smaller triangles is congruent to one of the other six smaller triangles.
Lets start by showing that the areas of ΔABF and ΔCBF are congruent. These triangles have the same altitude (or height) and congruent bases.
Using the formula, Area = ½(base)(height), we see that ΔABF ≅ΔCBF.
Thus, 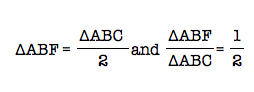
Now, lets show that 3(ΔAGF) = ΔABF. Since, A, G, and D lay on the same line, ΔAGF and ΔABF have the same altitude.

We know that the centroid is ⅔ the distance from a vertex to the midpoint of the opposite side.
Therefore, BG is ⅔ the length of BF and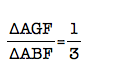
Hence,

The area of ΔAGF is ⅙ of the area of ΔABC.
Similarly, we can show that the areas of the remaining 5 remaining triangles are each equal to ⅙ the area of ΔABC, proving that the medians divide the triangle into 6 smaller triangles of equal area.

